
| n = - etrans / elongitudinal |
| e = DL/L. |
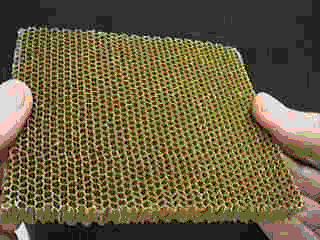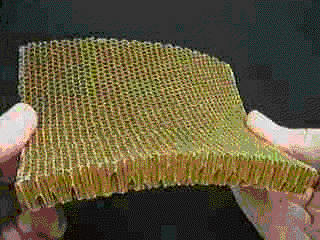
 In the structural view, the reason for the usual positive Poisson's ratio is that inter-atomic bonds realign with deformation. Stretching of yellow honeycomb by vertical forces, shown on the right, illustrates the concept. Negative Poisson's ratio in designed materials and in some anisotropic materials is by now well known.
In the structural view, the reason for the usual positive Poisson's ratio is that inter-atomic bonds realign with deformation. Stretching of yellow honeycomb by vertical forces, shown on the right, illustrates the concept. Negative Poisson's ratio in designed materials and in some anisotropic materials is by now well known.
|
n = (3K - 2G)/(6K + 2G)
E = 2G( 1 + n) E = 3K(1 - 2 n) |
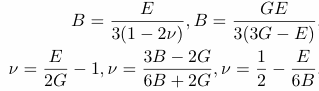
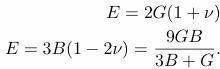
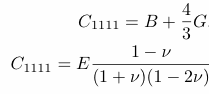
|
Material
Isotropic upper limit [1] Rubber [6] Indium [11] Gold [4] Lead [4] Copper [7] Aluminum [4] Copper [4] Polystyrene [6] Brass [1] Ice [8] Polystyrene foam [6] Stainless Steel [7] Steel [1] Tungsten [4] Tungsten Zinc [5] Fused quartz [9] Boron [12] Beryllium [4] Re-entrant foam [10] Isotropic lower limit [1] |
Poisson's ratio
0.5 0.48- ~0.5 0.45 0.42 0.44 0.37 0.34 0.35 0.34 0.33 0.33 0.3 0.30 0.29 0.30 0.28 0.25 0.17 0.08 0.03 -0.7 -1 |
References
[1] I. S. Sokolnikoff, Mathematical theory of elasticity. Krieger, Malabar FL, second edition, 1983. [2] A. M. James and M. P. Lord in Macmillan's Chemical and Physical Data, Macmillan, London, UK, 1992. [3] G.W.C. Kaye and T.H. Laby in Tables of physical and chemical constants, Longman, London, UK, 15th edition, 1993. [4] G.V. Samsonov (Ed.) in Handbook of the physicochemical properties of the elements, IFI-Plenum, New York, USA, 1968. [5] G. Simmons, and H. Wang, Single crystal elastic constants and calculated aggregate properties: a handbook, MIT Press, Cambridge, 2nd ed, 1971. [6] J. A. Rinde, Poisson's ratio for rigid plastic foams, J. Applied Polymer Science, 14, 1913-1926, 1970. [7] D. E. Gray, American Institute of Physics Handbook, 3rd ed., chapter 3, McGraw hill, New York, 1973. [8] E. M. Schulson, The mtructure and mechanical behavior of ice, JOM, 51 (2) pp. 21-27, 1999. article link [9] H. H. Demarest, Jr., Cube resonance method to determine the elastic constants of solids, J. Acoust. Soc. Am. 49, 768-775 (1971). [10] R. S. Lakes, Foam structures with a Negative Poisson's ratio, Science, 235 1038-1040, 1987. [11] D. Li, T. M. Jaglinski, D. S. Stone, and R. S. Lakes, Temperature insensitive negative Poisson's ratios in isotropic alloys near a morphotropic phase boundary, Appl. Phys. Lett, 101, 251903, Dec. (2012). [12] K. A. Gschneidner, Jr., Physical Properties and Interrelationships of Metallic and Semimetallic Elements, Solid State Physics, 16, 275-426, 1964 |
| (sxx/ exx)= E. |
| (sxx/exx)= C1111= E ((1 - n) / (1 + n)(1 - 2n)). |
 Practical example
- aircraft sandwich panels.
Practical example
- aircraft sandwich panels. Google bookmark
Google bookmark